pH lectures - introduction to the acid/base equilibrium
Most solutions containing different ions are in state of equilibrium - all concentrations are constant and not changing in time. This equilibrium is dynamic which means we have forward and reverse reactions going in the solution at the same rate, so that concentrations are not changing. This kinetic part of equilibrium is out of scope of our interest. We are going to concentrate our attention on the methods of finding the equilibrium in the solution being mixture of water, acid and base. Finding such equilibrium is always a part of pH calculation - even if we are often concentrated only on pH calculation, once we know pH we are usually able to calculate concentrations of all other ions. In fact we could as well concentrate our efforts on any other ion, but pH scale is very universal and knowing pH we can often tell a lot about the solution.
Let's start with several definitions that we will use extensively throughout the site. They are nothing else but the quantitative formulation of Le Chatelier's principle (that I am assuming you are familiar with).
Let's assume we have acid HA, base BOH, and their analytical concentrations (ie concentrations off all forms present in solution) are respectively Ca and Cb.
For the acid dissociation reaction
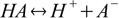
equilibrium is described by the acid dissociation constant defined as
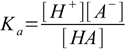 1.1
1.1For the base dissociation reaction
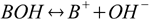
base dissociation constant is defined as
 1.2
1.2(you will find other ways of expressing the same reaction equilibrium in the other constants section).
Additional parameter used quite often to describe dissociation is a dissociation percentage (or dissociation fraction), defined as ratio of concentration of dissociated molecules to concentration of all molecules put into the solution. While not directly useful for finding equilibrium dissociation percentage is often used to validate assumptions done during calculations.
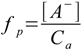 1.3
1.3It is commonly believed that strong acids and strong bases are fully dissociated. For most practical purposes this is true, but they have their dissociation constants as well and in some cases effects of partial dissociation can be observed.
Finally we will often need water ionization constant:
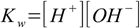 1.4
1.4pH and pOH defined in the pH definition section can be used not only as measure of the ions concentration, but also in calculations. Let's take logarithm of both sides of 1.4 equation:
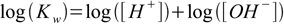 1.5
1.5Change signs and use p notation:
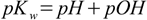 1.6
1.6Equation 1.6 is often used when we need to calculate pH but it is much easier to calculate pOH and vice versa.



